The International Renewable Energy Agency (IRENA) is developing an online Geographic Information System (GIS) to support scientists and decision makers in the development of renewable energy resources. The GIS forms the Global Atlas which as of 2014, has referenced more than 1000 datasets. These include geophysical quantities, topography, as well as population density, local infrastructure, land use and protected areas. The goal is to allow advanced energy and economic calculations for assessing the technical and economic potential of renewable energy.
The Atlas is divided into the chapters Wind, Solar, Geothermal, Bioenergy, Marine and Hydro. The Gravity maps are included in the chapter Geothermal energy. The gravity data have been derived from the GOCE satellite and are presented in two maps, the Free Air gravity map, and the Bouguer map. GOCE is part of the Earth Explorer family of earth observation satellites of the European Space Agency (ESA), see the GOCE earthnet page for more information.
The data can be superposed to other geothermal quantities as heat flow directly in the Atlas. Here the in depth information is given on calculation of the maps and the link to geothermal energy.
The Global Atlas initiative builds on a strong international partnership involving national institutes, energy agencies, private companies and international organizations for data and expertise promoting renewable energy. It started with solar and wind, and is progressively expanding to include further resources for bioenergy, geothermal and hydropower during 2014, and marine energies in 2015.
Figure 1: GOCE free air gravity disturbance. Calculation height=8000m above reference ellipsoid. Unit: mGal. Derived from averaging a full set of different observations of the satellite GOCE. Gravity disturbance field is obtained by subtracting the field of an ellipsoidal homogeneous Earth model with mass equal to the mass of the real Earth (GRS80 reference field). This field reflects mostly superficial density variations in the Earth’s crust.
Figure 2: GOCE Bouguer anomaly: calculation height =8000m above reference ellipsoid. Unit: mGal. This field differs from the GOCE free air disturbance by subtracting the effect of global elevated land masses and the effect of ocean basins filled with water. This field reflects to a great deal the thickness variations of the uppermost layer of the stratified earth, the crust. The crust has an average lower density than the underlying mantle, and therefore a thin crust produces an increased positive Bouguer anomaly. The greater amplitude of this signal masks the superficial geologic density variations seen in the free air gravity disturbance.
Usage of the gravity disturbance and Bouguer fields in geothermal applications
The Geothermal energy exploitation relies on access of increased temperature of the subsurface. The crustal temperature is governed by heat flux from the hot mantle and by heat production by radioactive decay in the crust. A thin crust brings the hotter mantle closer to the surface and therefore an increased temperature is found at shallower depth. A magmatic intrusion of molten rock is hot and conserves the heat until it slowly cools off inside the crust. In orogens the natural radioactivity of rocks produces heat, so an increase of heat which is independent from the mantle can exist. To bring the heat to the surface, water is the transport vehicle. Solid rock is too compact to have hot fluids reach the surface, so permeable paths must be found. The borders between different rock types generate permeable geometries forming the pathways. The essential components for exploiting the geothermal energy are presence of heat in the upper layers of the crust and the means to vehicle it to the surface for use to the benefit of society.
The measurement of heat inside Earth crust is a time
consuming task so indirect investigation methods are needed
that can be employed on a large scale. The gravity fields of
GOCE are a new investigation tool for large scale mapping.
The Bouguer map and the free air map give complementary
information. Generally speaking, thicker crust produces more
negative Bouguer values, thin crust more positive Bouguer
values. This is seen very clearly in the high Bouguer values
in the oceanic areas and negative Bouguer values for
mountain ranges. The free air field allows mapping of
geologic structures, from which borders separating rock
types are identified. Prospecting of geothermal heat sources
is time consuming and must be focussed to limited areas
which have a high probability of being exploitable. The
gravity maps from GOCE integrated with the heat flow values
and heat flow maps in the IRENA database form a tool which
allows a global probability classification, especially
useful in areas where no local gravity and seismic data are
available. Once a promising candidate area has been
identified, the successive step is local surveying involving
high resolution measurements and detailed investigations.
For illustration the heat flow variations for Italy (black
isolines in mW/sqm, deduced from Cataldi et al., 1995) are
overlain on the Bouguer anomaly in the database of IRENA and
the screenshot is shown in Figure 3. The highest heat
flow is found in the Tyrrhenian Sea, which correlates well
with the positive Bouguer values (orange color), and
reflects the thinned crust. The low Bouguer values in the Po
plain are not reflecting crustal thickening, as the
topography is low and flat in the Po plain, but density
variations in the subsurface, which we can better identify
in the gravity disturbance. The increased heat flow values
along the western coast of central Italy correlate to
increased gravity values, but are masked at the scale of the
present graph. In Figure 4 the heat flow values are overlain
on the gravity disturbance map. Here the full extent of the
Po basin is well seen by the low gravity values (blue) and
correlates with the low heat flow. The blue area of the
gravity extends into the Adriatic which shows how the
sedimentary units are traced beyond the area which can be
surveyed on the surface. Towards the Alps a slight increase
in heat flow values is observed which follows the isolines
of the Bouguer map and of the gravity disturbance. This
increase is generated by the greater amount of heat
production in the elevated topography due to the natural
radioactivity of the rocks forming the Alpine range. It can
be verified that the gravity maps delineate the different
domains of the heat flow and the directionalities of these
domains. Analogous considerations can be made when
investigating an area in which limited information on heat
flow is available and where a classification of the terrain
is needed. The steps involve critical analysis of the
gravity disturbance, the Bouguer field, the topography and
possibly a geologic map to formulate the extent of different
terrains and determine the focus areas for further
investigation.
Figure 3: Overlay of heat flow values in mW/sqm on Bouguer
gravity values. The highest heat flow is found in the
Tyrrhenian Sea and is seen well in the increased Bouguer
values. The low values in the Po plain correspond to lower
values in the Bouguer anomaly. Map produced on the IRENA
database.
Figure 4: Overlay of heat flow values in mW/sqm on gravity disturbance map. The gravity disturbance is low (blue values) over the entire Po basin and correlates very well with the full extent of low heat flow. Map produced on the IRENA database.
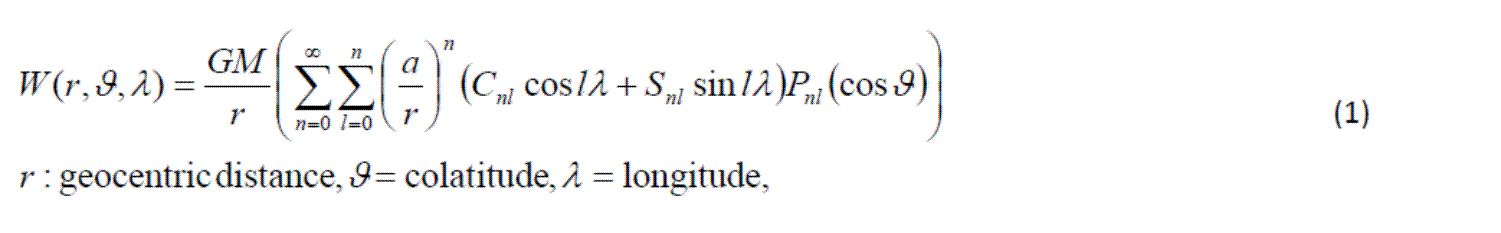
 Coefficients
of the spherical harmonic expansion of the gravitational
field.
Coefficients
of the spherical harmonic expansion of the gravitational
field.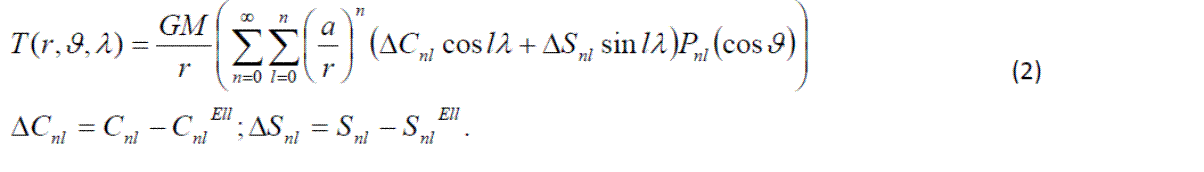
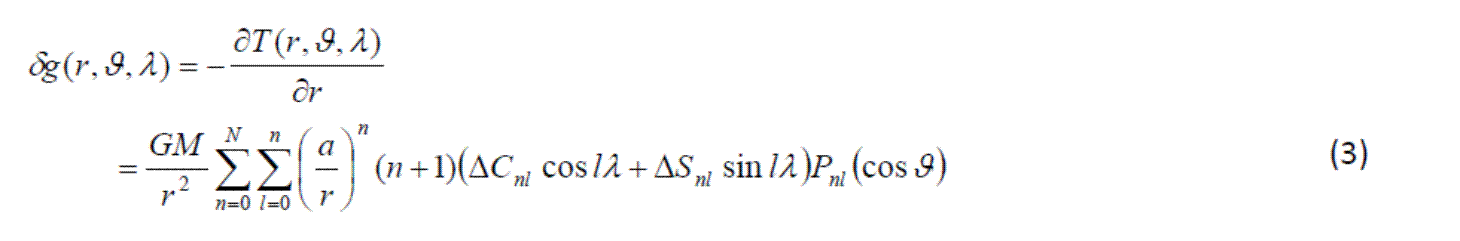
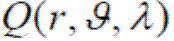 , expressed in
spherical harmonic expansion, the effect of topography is
calculated by:
, expressed in
spherical harmonic expansion, the effect of topography is
calculated by: 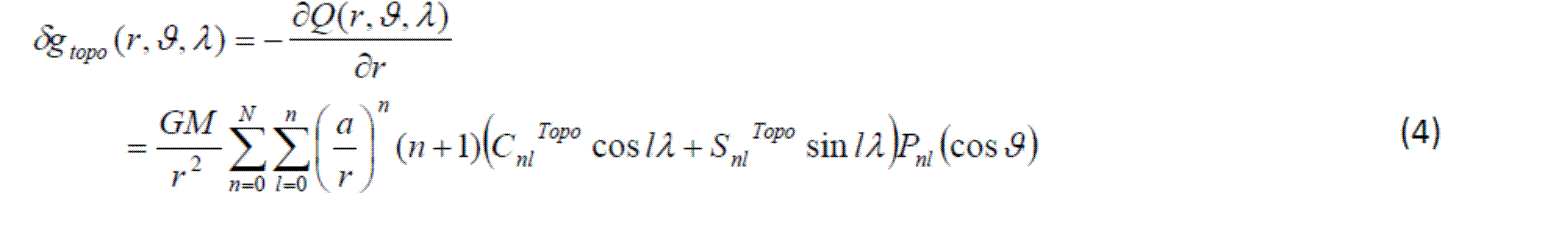
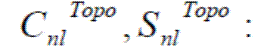 Coefficients
of the spherical harmonic expansion model of the gravity
potential of topographic masses.
Coefficients
of the spherical harmonic expansion model of the gravity
potential of topographic masses.